LaTeX templates and examples — Physics
Recent

Recientemente, el ser humano ha aprendido a obtener electricidad a partir de la energía solar mediante procesos fototérmicos y fotovoltaicos. Los primeros operan bajo principios semejantes a los de las centrales térmicas convencionales. Los denominados fotovoltaicos, presentan una importante simplificación respecto a los procesos energéticos usuales, debido a que transforman una energía primaria, la solar, en electricidad de un modo directo, es decir, sin transformaciones intermedias en otras formas de energía. Estos dispositivos son estáticos y en absoluto semejantes a las generadores convencionales. Los procesos que implican la dispersión o absorción de radiación por materia son tres, a saber: el \textbf{efecto fotoeléctrico} (proceso involucrado en la conversión de energía solar en energía eléctrica), el \textbf{efecto Compton} y la \textbf{producción de pares}. El crecimiento exponencial es probablemente el mas familiar para los ingenieros electricistas, en la ecuación del diodo, la cual relaciona la corriente en el diodo $I$, al voltaje en el diodo $V$ \begin{equation}\label{eq:1} I=I_0\left(e^{\frac{qV}{kT}}-1\right) \end{equation} Mientras la ecuación \eqref{eq:1} es fundamental en el rendimiento de las celdas fotovoltaicas, muchos otros procesos físicos son caracterizados por el crecimiento exponencial.\\ La celda fotovoltaica es un diseño especial de la \textit{unión p-n} o diodo de barrera de Schottky. En una célula solar, la luz solar entrante desaloja electrones y sus contrapartes cargadas positivamente, llamados huecos, en la capa media del dispositivo. Los electrones luego migran a un electrodo, liberando alguna carga eléctrica y vuelven a los huecos a través de otro electrodo para completar el circuito \subsubsection*{Fundamentos de Dispositivos Fotovoltaicos} \begin{enumerate} \item \textit{Generación de carga}: La luz excita los electrones, liberándolos para moverse a través del cristal. \item \textit{Separación de la carga}: Un campo eléctrico dirigido hacia el material (unión p-n) barre electrones. \item \textit{Colección de carga}: Los electrones depositan su energía en una carga externa, lo cual completa el circuito. \end{enumerate}
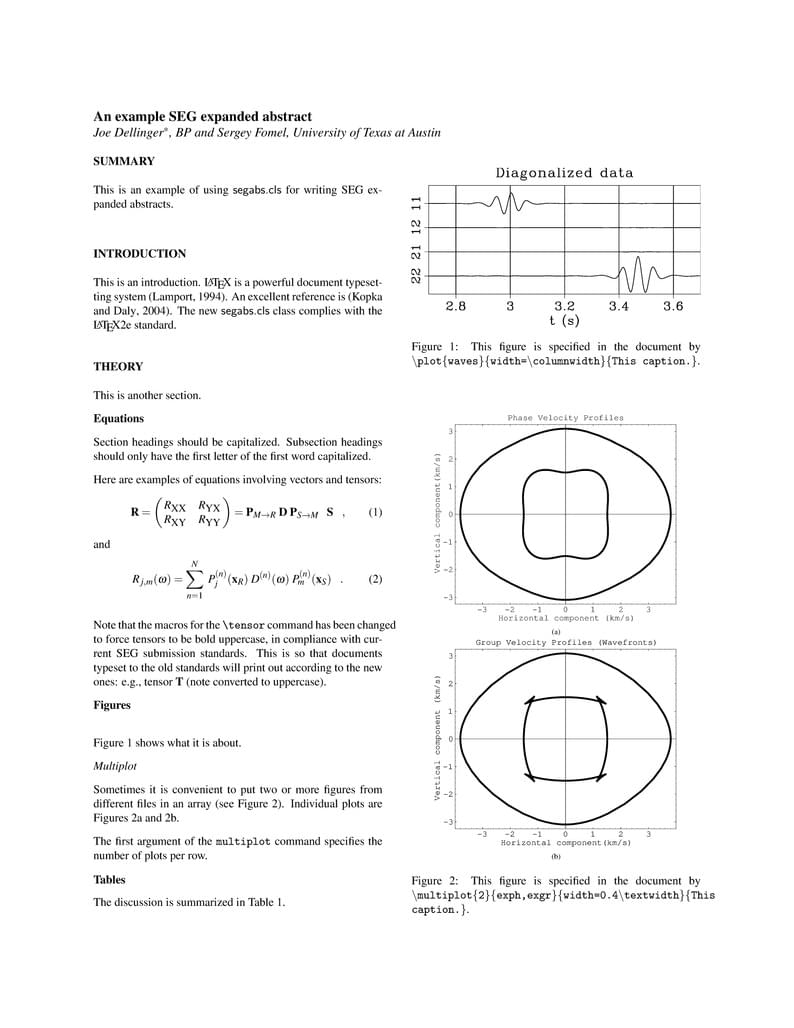
Template for writing SEG abstract.

GEOPHYSICS, published by the Society of Exploration Geophysicists since 1936, is an archival journal encompassing all aspects of research, exploration, and education in applied geophysics. This is the LaTeX template for GEOPHYSICS papers.
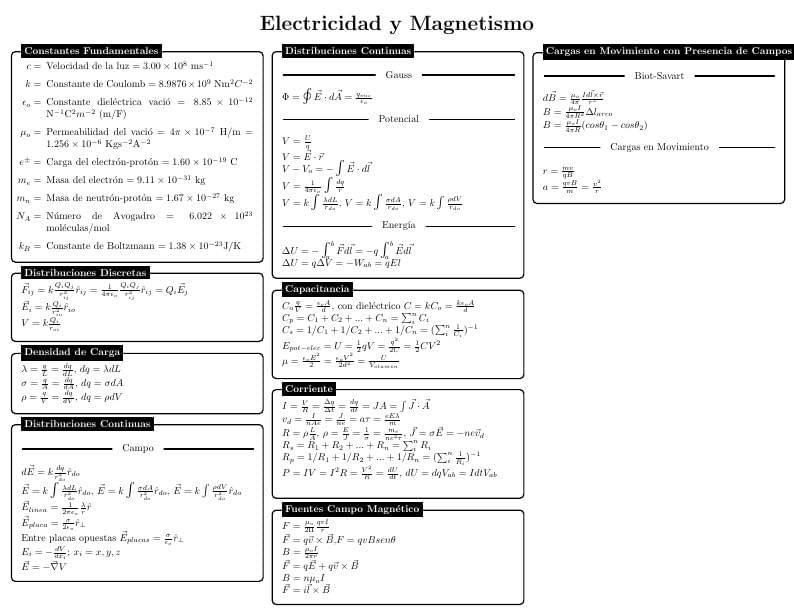
Herramienta para generar hojas de ayuda para Física
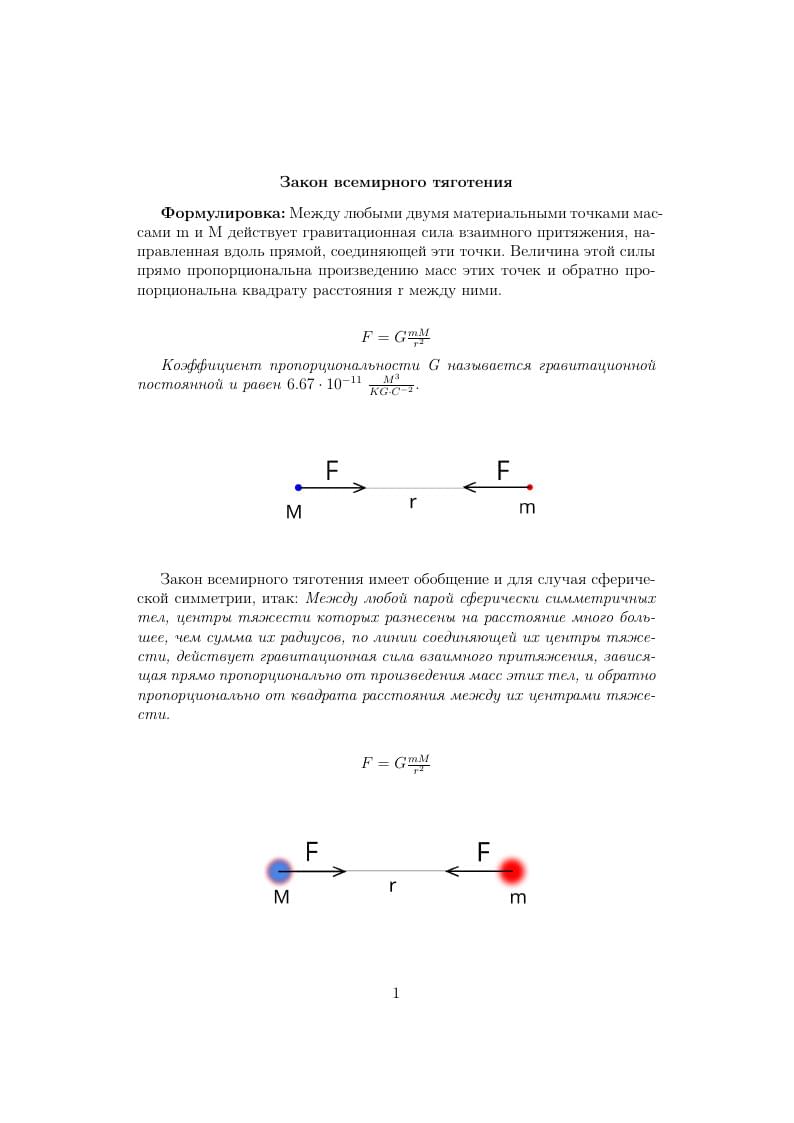
Параграф для энциклопедии, по соответствующей теме.

informe de practica pendulo fisico

This is the template used in Physics 211 (calculus-based mechanics) as taught at Cortland High School in Cortland, NY. The abstract should be a short 3-5 sentence paragraph. In it, you should state the hypothesis, what you did, and what you found. The abstract is meant to be a very short summary of your paper to follow. It is a good suggestion to write the abstract last in your report after you have written everything else. This will allow you to best summarize your work.

Guía de presentación de trabajos

Sinds enkele jaren ben ik op zoek naar eenvoudige wiskundige en fysische problemen die onverwacht gerelateerd zijn met het getal \(\pi\). In The bouncing balls and pi beschreef ik eerder al hoe de opeenvolgende decimalen van \(\pi\) kunnen berekend worden door twee ballen volledig elastisch tegen elkaar en tegen een muur te laten botsen. In dit artikel zal ik aantonen hoe het getal \(\pi\) tevoorschijn komt door een oneindige serie rechthoeken met oppervlakte 1 spiraalsgewijze aan elkaar te kleven. In een veralgemening van dit probleem duikt op een natuurlijke wijze de gammafunctie en de formule van Stirling op.
\begin
Discover why over 20 million people worldwide trust Overleaf with their work.